(119i) Diffusion of Interacting, Nonionic Micelles with Hydrophobic Solutes

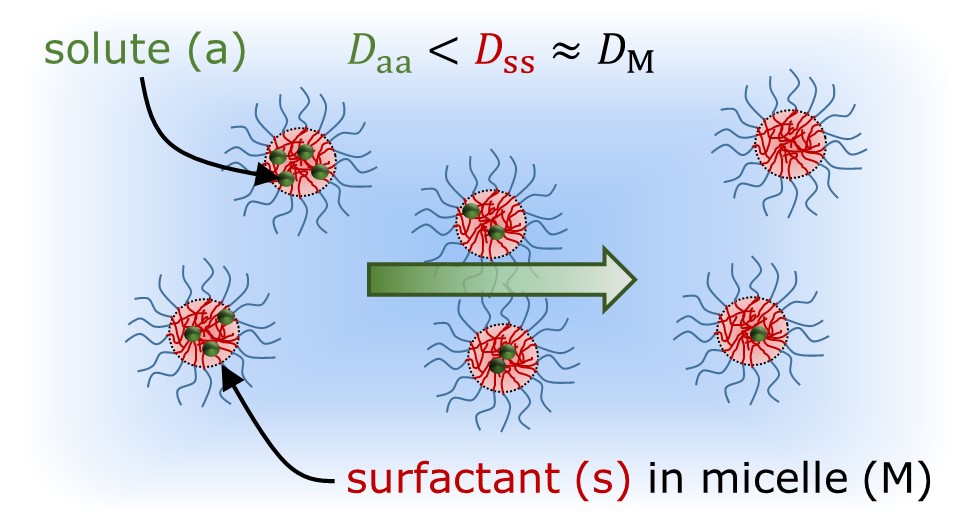
Taylor dispersion and dynamic light scattering techniques were used to measure the ternary diffusion coefficient matrix [D] and the micelle gradient diffusion coefficient, respectively, in crowded aqueous solutions of decaethylene glycol monododecyl ether (C₁₂E₁₀) with either decane or limonene. The results indicate that C₁₂E₁₀ diffused down its own gradient with the micelle gradient diffusivity while the hydrophobic solute diffused down a solute gradient at a much slower rate. Furthermore, strong diffusion coupling, comprising solute diffusion down a surfactant gradient and surfactant diffusion up a solute gradient, was also observed, with cross diffusivities that were on the order of or larger than the main diffusivities. Measurements of the average micelle aggregation number, hydration index, and hydrodynamic radius, obtained using both static and dynamic light scattering methods, indicate that solute-containing micelles interacted as hard spheres with an average radius and aggregation number that increased linearly with the molar ratio of solute to surfactant for both hydrophobic solutes. A theoretical model, developed using Batchelor’s theory for gradient diffusion in a polydisperse system of interacting hard spheres, was effectively used to predict [D] with no adjustable parameters. Comparison with theory indicates that solute diffused down its own gradient by micelle self diffusion while surfactant diffused down a surfactant gradient by micelle gradient diffusion. It is also shown that intermicellar interactions drove solute diffusion down a C₁₂E₁₀ gradient by a volume exclusion effect while an increase in the average micelle aggregation number and hydrodynamic radius with molar ratio along the diffusion pathway was necessary to drive surfactant diffusion up a solute gradient.