(256e) CFD and Experimental Study of Gas Holdup and Liquid Velocity in an Internal-Loop Airlift Reactor with Newtonian and Non-Newtonian Fluids
AIChE Annual Meeting
2020
2020 Virtual AIChE Annual Meeting
North American Mixing Forum
Mixing in Rheologically Complex Fluids and Polymeric Systems
Tuesday, November 17, 2020 - 8:45am to 9:00am
Unsteady three-dimensional flow induced by a type-L diffuser installed inside an airlift reactor agitating Newtonian and non-Newtonian (shear-thinning) fluids was simulated. The Euler-Euler model was used in conjunction with the standard k-e model. Liquid circulation velocities (in the riser and downcomer sections), and gas holdup were numerically and experimentally evaluated as a function of the air inlet-velocity. Bubbles diameters were obtained from imaging capture. Obtained numerical results are in good agreement with experimental measurements.
1. Introduction
Airlift reactors (ALRs) are widely used in chemical and biochemical industrial processes. These are devices agitated by a continuous gas-phase in the form of bubbles that break-up within the liquid-phase, inducing an isothermal expansion promoting homogeneity inside the reactor [1]. ALRs can be classified into internal or external-loop reactors according to its geometry and the defined cyclic pattern through a loop, which divides the bioreactor into two zones: riser and downcomer.
Liquid circulation velocity (LCV), and gas holdup are considered key design parameters for ALRs. LCV influences mixing and mass transfer rates; whereas gas holdup is an index for the gas-phase mean residence time [2]. These parameters have been widely studied for Newtonian-fluids, however, in laboratory-scale and industrial applications, non-Newtonian (shear-thinning) fluids are frequently employed. Shear-thinning behavior brings uncertainty to the design, scale-up and operation of ALRs [3]. Experimental studies have been carried out on ALRs containing these working fluids [3-6]. Empirical correlations have been generated for this case, allowing to obtain the previously mentioned parameters.
Current interest in estimating global and local parameters for proper design and scale-up of ALRs is increasing. Global parameters can be obtained from conventional techniques. LCV has been measured by pulse injection-response, whereas gas holdup has been estimated by differential pressure measurements [7]. Conversely, measuring local values, e.g., shear-rate and its corresponding shear-stress and turbulence intensity, is rather complex, thus, experimental techniques (Laser Doppler velocimetry, LDV, and Particle Image Velocimetry, PIV) have been used in order to determine both global and local parameters. Although possible to obtain good results with these techniques, they have limitations for the fact that they demand specific experimental characteristics; mainly from the liquid phase (e.g., transparent fluids) and are normally expensive to attain [8]. On the other hand, the hydrodynamics induced in ALRs agitating Newtonian-fluids has been studied by means of Computational Fluid Dynamics (CFD) techniques [7, 9]. CFD enables knowledge-gathering based on the numerical simulations of the hydrodynamics at relatively low costs, enabling it as an excellent alternative for the design, scale-up, and performance-assessment for these devices.
It is clear that numerical studies for determining global and local parameters of ALRs agitating non-Newtonian-fluids are needed. The aim of this work is to determine both LCV and gas holdup within an ALR agitating both Newtonian and non-Newtonian (shear-thinning) fluids resorting to CFD techniques.
2. Methodology
2.1. Experimental
The internal-loop ALR employed was built out of glass with an internal diameter of 7.2cm. It is comprised with two main parts: 1) a gas L-diffuser, and b) a draft tube. The drilled-diffuser included five 0.1 cm diameter holes and was situated at 1.2cm from the bottom. The draft tube dimensions considered were: 4.2, 4.5 and 20cm for inner diameter, outer diameter, and high, respectively. The liquid height was set at 26.0 cm, i.e. a liquid volume of 1L. Tap water and two aqueous solutions of Carboxy-Methyl-Cellulose (CMC) at 0.25 and 0.50%wt/v, respectively were used as working fluids, while air was used as gas phase. The apparent viscosity of the shear-thinning CMC solutions were measured with a viscometer. The data obtained was then fitted to a power-law model [5]:
η = τ/γ =k(γ)n-1 , (1)
where k and n are the consistency index and the flow index, respectively. Values for k, n and density are provided in Table 1.
Both the gas holdup (εG) and LCV were evaluated considering five air inlet-velocities: UGI=94.3, 141.5, 188.6, 235.8 and 282.9cms-1, then εG was calculated from the difference between the volume of the aerated and non-aerated liquid-phase [5]:
εG = (Ha-Hna)/Ha, (2)
where Ha and Hna are the aerated and non-aerated liquid heights, respectively. The LCV was determined by using a chronometer and calculating the displacement time of sodium-polyacrylate spheres in riser (Δx=180mm) and downcomer (Δy=120mm). Bubble diameters were calculated by image capturing in situ in addition with the WebPlotDigitizer software.
2.2. Numerical simulations
To simulate the fluid flow, a CAD mesh comprised by hexahedrons was built in ANSYS-Meshing®. This mesh consisted of 251264 cells and skewness lesser than 0.85. The numerical solution was obtained using ANSYS-Fluent® 17.1. The transitional Euler-Euler model alongside with the Grace drag-model was used. Turbulence was modeled considering the standard k-ε model. Non-slip boundary conditions were applied to all solid surfaces. Atmospheric pressure conditions were defined at the free surface of the liquid column. Coupled pressure-velocity was calculated by using the Phase-coupled simple scheme. Spatial discretization was achieved employing the second order upwind scheme. Momentum and volume fraction were approximated by the QUICK scheme. Turbulence parameters and time discretization were obtained employing the first order upwind scheme. Time steps of 10-3 s were considered.
3. Results
Table 2 shows bubble-diameter as a function of UGI and CMC concentrations. It can be seen that increasing both UGI and CMC simultaneously results in a bubble-diameter increase. These results are in agreement with those reported by Deng et al. [4], who attribute this effect to the fact that increasing liquid viscosity led to a decreasing in turbulence intensity, and therefore, the bubble break-up rates are lower than their coalescence.
Figure 1 exhibits both experimental and simulation values for LCV and gas holdup, determined as a function of UGI and CMC concentration. The LCV both in riser (ULR) and in downcomer (ULD) are shown in Figures 1a) and b), respectively. For both regions, LCV increases with UGI (due to the increase in pressure drop between the riser and downcomer) and decreases when the CMC concentration increases (which is caused by an increase in the flow resistance). Popovic et al. [6] reported similar results. Average relative differences of 7.3% and 16.7% between experimental and numerical velocity values in the riser and the downcomer were obtained in the present study. Results for gas holdup are showed in Figure 1c), these exhibits an increase in εG when UGI and CMC are increased. This behavior can be attributed to fact that when liquid viscosity is increased, bubble rising-velocity decreases (results no reported in this work), thus leading to longer bubble residence times resulting in higher gas holdup. In this case, an average relative difference between experimental and numerical gas holdup of 9.0% was obtained.
Conclusion
Global parameters for an internal-loop airlift reactor such as LCV and gas holdup were accurately predicted by the employment of CFD simulation techniques. Future studies can take advantage of this techniques by allowing for parameter-prediction without resorting to the assembly of the real devices.
References
[1] M. A. Lizardi-Jiménez, G. Saucedo-Castañeda, et al., Chemical Engineering Journal 2012, 187160-165.
[2] W.-J. Lu, S.-J. Hwang, et al., Chemical Engineering Science 1995, 50(8), 1301-1310.
[3] Y. Kawase and N. Hashiguchi, The Chemical Engineering Journal and the Biochemical Engineering Journal 1996, 62(1), 35-42.
[4] Z. Deng, T. Wang, et al., Chemical Engineering Journal 2010, 160(2), 729-737.
[5] M. Gavrilescu and R. Z. Tudose, Bioprocess Engineering 1997, 18(1), 17-26.
[6] M. Popović and C. W. Robinson, Biotechnology and Bioengineering 1988, 32(3), 301-312.
[7] H.-P. Luo and M. H. Al-Dahhan, Chemical Engineering Science 2008, 63(11), 3057-3068.
[8] E. M. Marshall and A. Bakker, Handbook of industrial mixing: science and practice 2004, 257-343.
[9] M. Å imÄÃk, A. Mota, et al., Chemical Engineering Science 2011, 66(14), 3268-3279.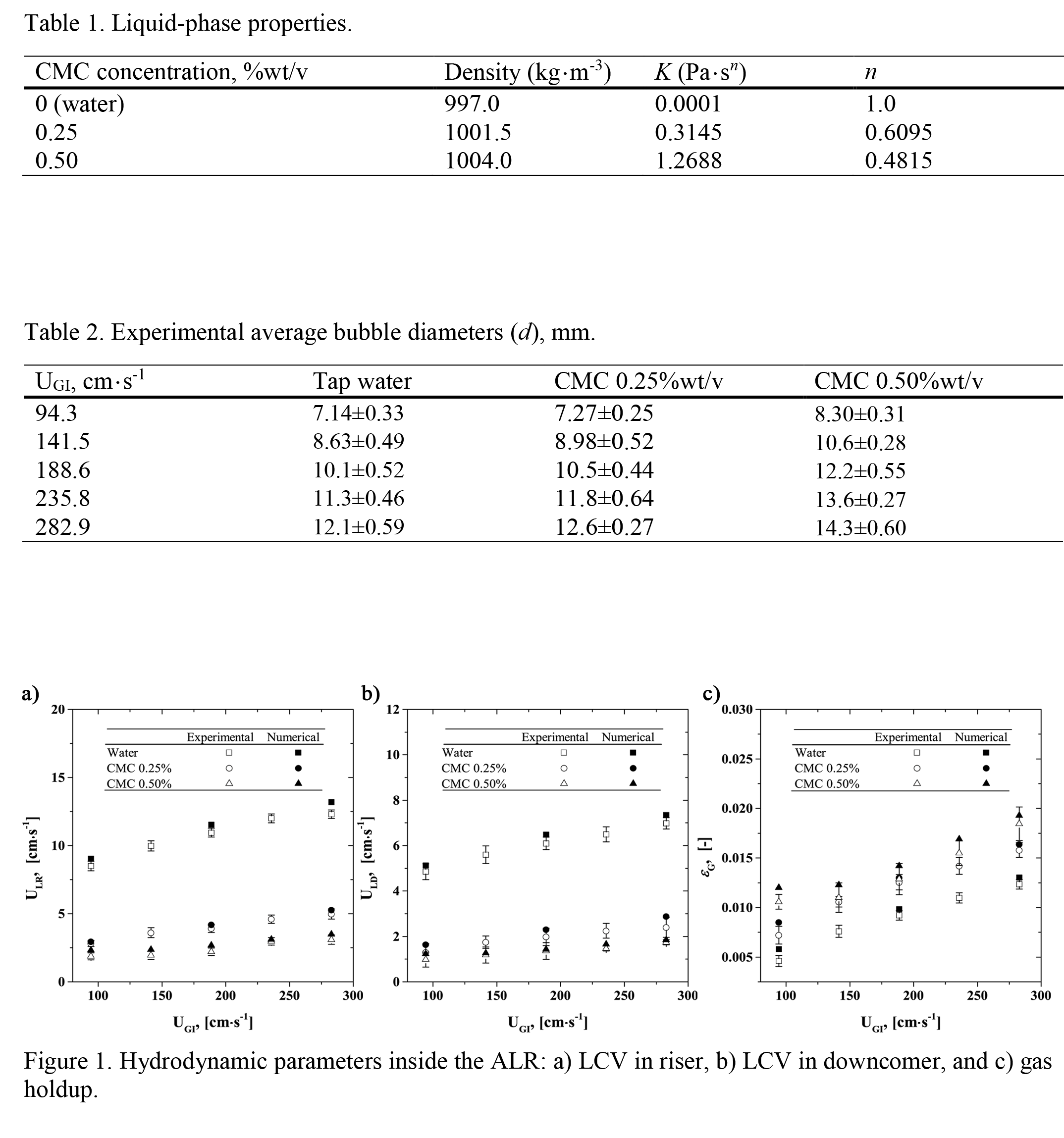
Topics
Checkout
This paper has an Extended Abstract file available; you must purchase the conference proceedings to access it.
Do you already own this?
Log In for instructions on accessing this content.
Pricing
Individuals
| AIChE Pro Members | $150.00 |
| AIChE Emeritus Members | $105.00 |
| AIChE Graduate Student Members | Free |
| AIChE Undergraduate Student Members | Free |
| AIChE Explorer Members | $225.00 |
| Non-Members | $225.00 |
