(576h) Continuous Solid-State Deracemization Via Temperature Cycles – a Model-Based Process Development

Although preferential crystallization is a standard industrial process to obtain enantiopure crystals of conglomerate forming compounds, its maximum theoretical yield is 50%, unless one adds to it recycle and racemization steps [1]. On the contrary, one can produce an enantiopure powder from an initially racemic one with 100% yield through solid-state deracemization by exploiting several techniques [1,2]. Among such techniques, one based on temperature cycling is a promising candidate for industrial applications due its simplicity of operation [1,3,4]. However, the process itself is complex and hence it warrants the need for a mathematical model to understand the effect of initial conditions and of operating parameters on the process outcome, i.e. the production of the desired enantiomer, the process time, etc.

Continuous processes have been shown to outperform batch operation. In addition, they can recover from process disturbances, because they run at stable steady state [5]. Continuous crystallization processes have been adapted for the separation of conglomerate forming enantiomers, in order to exploit the advantage of continuous crystallization techniques. In case racemization of enantiomers in the solution proves difficult, continuous preferential crystallization without a racemization reaction is a widely studied choice [6]. If the racemization reaction is available, continuous preferential crystallization with racemization reaction or continuous Viedma ripening can be performed. Alternatively, deracemization via temperature cycles in mixed suspension mixed product removal crystallizers (MSMPRC) can also be performed, but this is an unexplored method of choice.

Mathematical model

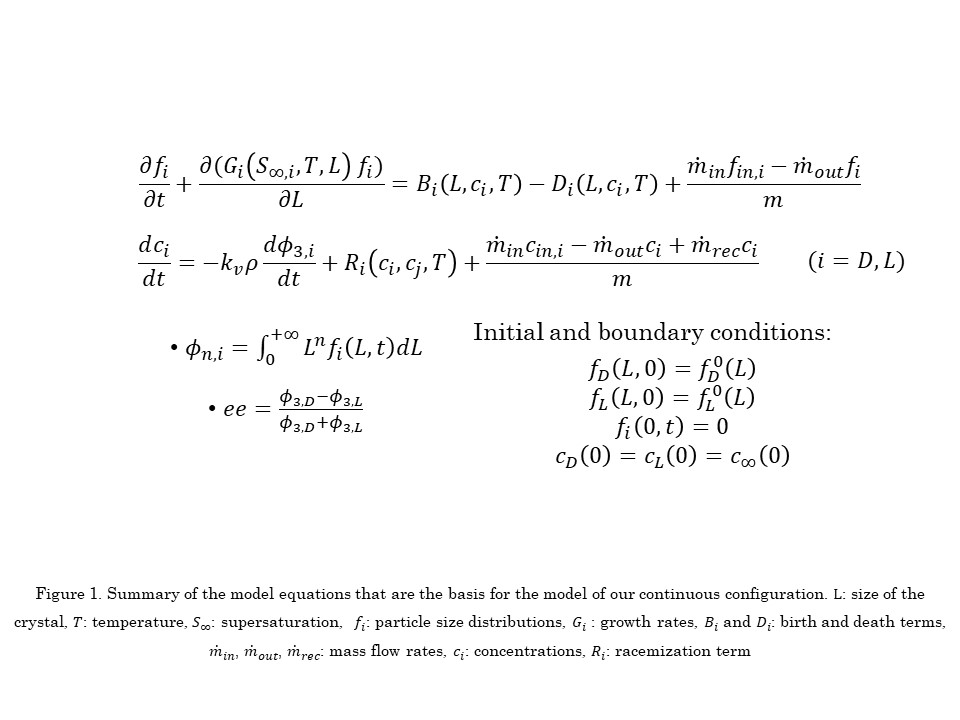
A population balance equation (PBE) -based mathematical model has been developed to provide a clear explanation of the phenomena involved in the deracemization process. The model describes the interplay of several phenomena involved in a temperature cycling process (size-dependent solubility, crystal growth and dissolution, agglomeration, attrition/secondary nucleation and racemization) accounting for the dependence of their thermodynamic and kinetic parameters on the crystal size and on the temperature [5].

Results and discussion- batch

Using the PBE-model that we have introduced, first, we have simulated temperature cycles in batch crystallizers. A mathematical model of deracemization is a useful tool to perform process analysis: it provides information on all the relevant physical quantities during the whole process and enables decoupling of the individual phenomena, and investigating the effect of variations in e.g., the breakage rate intensity, the agglomeration intensity, etc. After identifying the necessary phenomena to deracemize using temperature cycles, process characterization was performed using the developed model, in order to identify an optimal process. Parametric studies varying operating parameters like the temperature and cooling rate were performed and based on the outcome it is shown that the simulation outcome qualitatively describes experimental results [1,4].

Moreover, by investigating the effect of slight variation in the initial conditions, an explanation to the large variations in deracemization time and in process outcome — that are typically observed in experiments — is provided using the mathematical model.

Results and discussion- continuous

The inherent disadvantage of the batch crystallization process is that it suffers from batch-to-batch product variability and requires control techniques to obtain the product with the specific quality of interest. As tuning the many degrees of freedom blindly while performing the actual experiments is ill-advised [7], this contribution aims at providing a mathematical tool that can be used to design robust continuous processes.

Therefore, we have extended our batch non-isothermal population balance-based model of solid-state deracemization via temperature cycles to simulate the proposed continuous configuration. To avoid undesired nucleation of the counter enantiomer, different process variants are considered, e.g., utilization of recycle loop, wet mill, fines dissolution loop. We have investigated the steady state operation of temperature cycles carried out in MSMPRC. Periodic operation of the continuous crystallizer via temperature cycling affects the transient behaviour of the process and thereby the attainable steady state. We have run simulations to study the interplay of the cycle time and the residence time with respect to the physical properties of the system, i.e., how the process characteristic times compare to the characteristic times of growth, dissolution and racemization.

The aim of this analysis was to verify whether one of the continuous configurations envisaged performs better than the batch alternative. The effect of the initial conditions (variations in the initial enantiomeric excess, in the suspension density, etc.) and of the operating parameters on the periodically perturbed process and its steady state in terms of productivity and attainable enantiomeric excess are identified. Together with this assessment, we aim to provide strategies for the optimal implementation of the process.

Acknowledgments

This research has received funding as part of the CORE project (October 2016 – September 2020) from the European Union’s Horizon 2020 research and innovation programme under the Marie Sklodowska-Curie grant agreement No 722456 CORE ITN.

References

[1] Breveglieri, G. M. Maggioni, M. Mazzotti, Cryst. Growth Des. 18 (2018) 1873-1881.
[2] L. Noorduin, W. J. P van Enckevort, H. Meekes, B. Kaptein, R. M. Kellogg, J. C. Tully, J. M. McBride, E. Vlieg, Angew. Chem., Int. Ed. 49 (2010) 8435-8438.
[3] Iggland and M. Mazzotti, Cryst. Growth Des. 18 (2011) 4611-4622.
[4] Suwannasang, A. E. Flood, C. Rougeot, G. Coquerel, Org. Process Res. Dev. 21 (2017) 623-630.
[5] Köllges and T. Vetter, Cryst. Growth Des. 17 (2017) 233-247.
[6] Vetter, C. L. Burcham, M. F. Doherty, AICHE J. 61 (2015) 2810-2823.
[7] Bodák, G.M. Maggioni, M. Mazzotti, Cryst. Growth Des. 18 (2018) 7122-7131.