(308f) High-Viscosity Cavitating Flow-through Vortex-Based Hydrodynamic Cavitation Device: Pressure Drop, Inception and Flow Characteristics

Hydrodynamic cavitation gaining significant attention due to its extensive applications, ranging from food and pharmaceutical processing to wastewater treatment. Vortex-based hydrodynamic cavitation (HC) devices provide several advantages over conventional lienerflow devices (e.g., orifice and venturi) for example, Early inception, reduced erosion, and larger cavitational yield. Recently, several authors investigated the role of the vortex-based HC device in different chemical processes e.g., wastewater treatment¹, reactions², emulsification³, etc. The internal flow dynamics inside the vortex-based HC device in terms of flow behaviour and cavitation dynamics were investigated for different device pressure drop and throat diameters. The overall flow behaviour and cavitation inception are strongly dependent on the viscosity of the liquid system. Despite several efforts in the experimental investigations and computational modelling of vortex-based HC devices, the study on the flow behaviour and cavitation dynamics in presence of highly viscous liquids are not available in the open literature. Therefore, The development of an experimentally verified modelling framework for explaining the behaviour of vortex-based HC devices is crucial for enhancing the applicability of HC devices in a wide range of chemical processes and reactions. The present work focuses on (i) experimental investigation of internal flow behaviour and cavitation inception in presence of high viscous gycerol+water mixture using a vortex-base HC device and (ii) development of multiphase Eulerian CFD models to simulate cavitating flow. The most important dimensionless numbers related to hydrodynamic cavitation, i.e., Euler number (Eu) and Reynolds number (Re) were analysed for different viscosities.

Experiments and Modelling

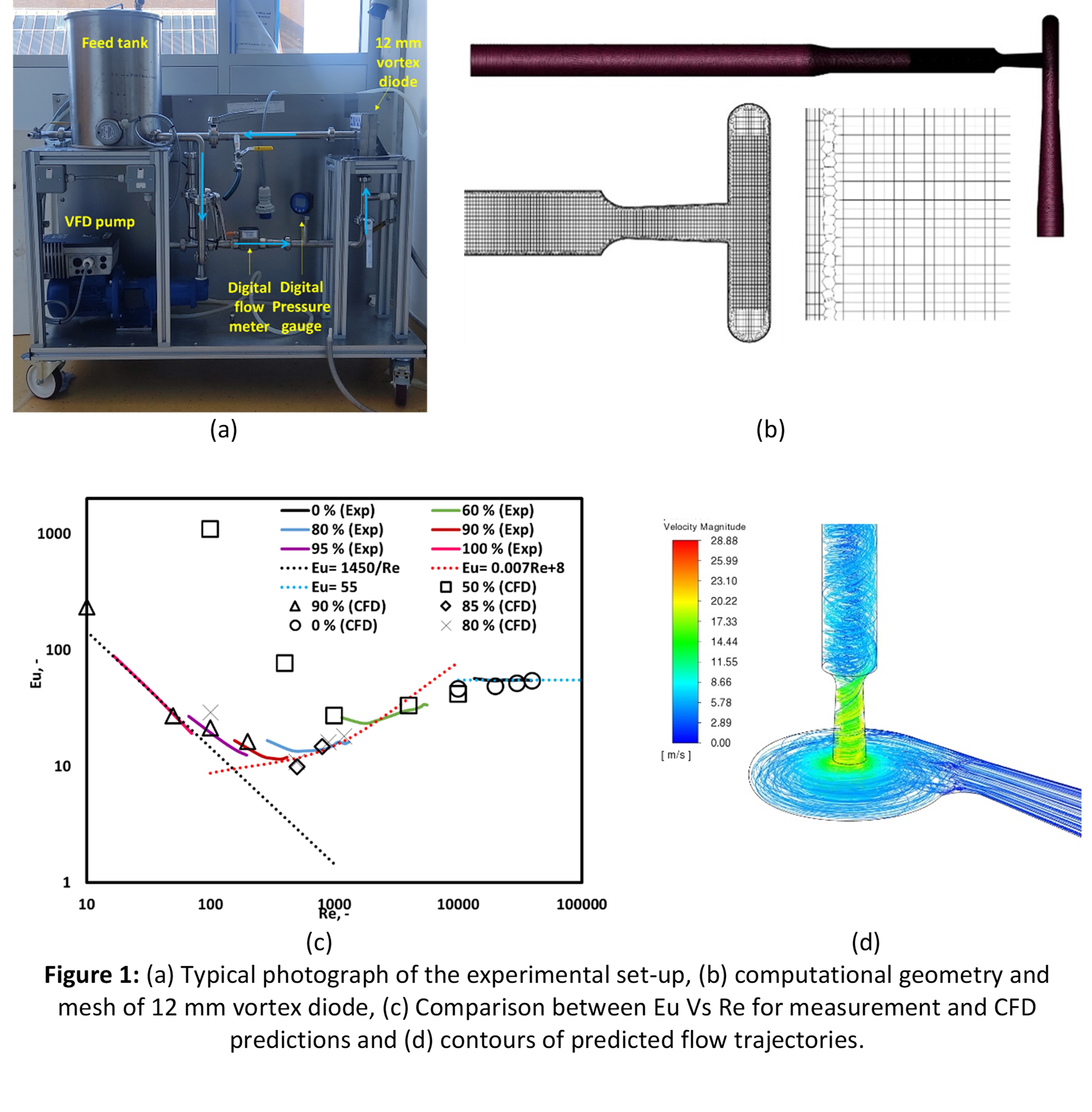
An attempt has been made to perform hydrodynamic cavitation experiments with different solution viscosities using a vortex-based HC device. The typical photograph of the experimental setup is shown in Figure 1a. The throat diameter of the cavitation device was 12 mm. To visualise the flow behaviour and cavitation inception, the experiments were performed using a transparent (PMMA) diode. Demineralised water (= 997 kg/m³, = 1.17 × 10^–3 Pa.s) was used as a liquid phase and the viscosity of water was altered by adding Glycerol (= 1261 kg/m³, = 1.412 Pa.s; ReAgent, UK) in the water. The experiments were performed with a total volume of 8 L with different glycerol percentages (0 – 100%). The pressure drop in the HC system is maintained in the range of 30 - 400 kPa. The present work is initiated to investigate the effect of viscosity on the internal flow behaviour, cavitation dynamics and overall device performance. A further attempt will be made to investigate the effect of device scaling and its consequences on the overall performance in terms of cavitation intensity and flow dynamics.

To understand the complex liquid-liquid multiphase cavitating flow field developed in vortex-based HC devices, transient CFD simulations were performed using the Eulerian mixture model in Ansys Fluent 2020 R1. The phase change due to the cavitation was modelled using Singhal et al.⁴ cavitation model. The k−ω SST model was adopted for turbulence modelling. The CFD results were used to quantitatively relate predicted pressure drop, velocity and energy dissipation rates required for the droplet breakage with the measurements. The mesh for the numerical solution was created with the Ansys MOSAICTM technology (see Figure 1b). Most of the fluid domain was filled with hex-core elements. The boundary layer with a first height of 0.02mm, 7 layers, and a 1.4 growth rate was created to maintain the maximum y+ < 10. The hex-core elements were connected with the Prizm layer with one layer of polyhedral elements. Necessary grading in critical areas was also given with the help of the body of influence. All the mesh attributes were maintained according to accepted standards. Figure 1b shows an overview of the computational geometry and mesh of the vortex-based HC device.

Key Results and Conclusions

The vortex-based HC experiments were performed for different viscosities (i.e., 1.17, 9.5, 61.5, 208, 388 and 866 cp) corresponding glycerol percentage of 0, 60, 80, 90, 95, 100% (see Figure 1c). A comparison was made between the Eu and Re to analyse the effect of viscosity on throat velocity, pressure drop and cavitation inception (see Figure 1c). Due to the lower viscosity (1.17 cp) in the case of a pure water system (0 % glycerol), the flow was highly turbulent. This led to higher swirl velocities inside the swirling chamber and resulted in cavitation inception at an even lower inlet pressure drop (∆P= 30 kPa). On the other hand at higher viscosity (866 cp; at 100% glycerol), the flow was dominated by the viscous force and as a result, the cavitation inception was suppressed due to the absence of adequate vortex flow. Two different regions can be seen in Figure 1c, (i) the laminar region, where the Eu was significantly influenced by Re and (ii) transient to turbulent, where Eu was moderately (in transient) and marginally (in highly turbulent) influenced by Re. The overall trend was predicted in three different zones and is shown in Figure 1c. The cavitation inception was found to be around Re of 350. Therefore, it is recommended to operate the vortex based HC device for the liquid systems which have viscosities up to 200 cp to get good performance of the device. The prima facie simulation performed on the HC device also predicted an Euler number similar to that of experimental results. The flow trajectories inside the vortex based HC device are shown in Figure 1d. Simulations to determine cavitation inception numerically are ongoing.

The measurements, models, devices, and strategies handling high-viscous systems and understanding flow behaviour and cavity dynamics in the present work will provide a sound basis for harnessing hydrodynamic cavitation for several chemical process applications (e.g., pharmaceutical, personal care, healthcare, food and paint industry). The devices, models and results presented in this work will pave the way toward realising the next-generation manufacturing platforms.

Acknowledgement

The authors greatly acknowledge the support provided by Ansys for a CFD license under an academic partnership programme. The authors also wish to acknowledge the Irish Centre for High-End Computing (ICHEC) for the provision of computational facilities and support.

References:

1. Ranade, V. V., Kulkarni, A. A. & Bhandari, V. M. Vortex diodes as effluent treatment devices: US Patent 9422952. (2016).
2. Sarvothaman, V. P., Simpson, A. T. & Ranade, V. V. Modelling of vortex based hydrodynamic cavitation reactors. Chemical Engineering Journal 0–1 (2018) doi:10.1016/j.cej.2018.08.025.
3. Thaker, A. H. & Ranade, V. v. Drop breakage in a single‐pass through vortex‐based cavitation device: Experiments and modeling. AIChE Journal 1–19 (2021) doi:10.1002/aic.17512.
4. Singhal, A. K., Athavale, M. M., Li, H. & Jiang, Y. Mathematical Basis and Validation of the Full Cavitation Model. Journal of Fluids Engineering 124, 617 (2002).