(308g) Shallow Water Analysis and Numerical Simulation of Laminar Planar Hydraulic Jump in Bingham Plastic Flow through an Open Rectangular Channel

Hydraulic jump is an intricate fluid flow phenomenon, associated with flow transition from supercritical (Froude number, Fr >1) to subcritical condition ( Fr <1), where Froude number ( Fr) is expressed as, Fr=V/√(gh) , V is the velocity of flow, g is acceleration due to gravity and h is depth of flow. The phenomenon is manifested as a sudden increase in liquid free surface height accompanied with significant loss of energy of the system. It is often used for flood controlling, energy dissipation associated with hydraulic structures and is also common in chemical process industries involving mixing of components, water treatment processes etc. Recently, planar hydraulic jump in thin film laminar flow, also known as ‘natural jump’ [1], has received considerable attention due to its wide applications in film draining, film coating, thin-film chemical reactors etc. However, most of the studies are reported for Newtonian liquids. Planar jumps in non-Newtonian liquids have rarely been investigated. The present investigation proposes a detailed study of planar hydraulic jump during laminar flow of Bingham plastic liquid over a horizontal rectangular open short channel.

Bingham plastic fluids do not deform as long as the shear stress (Ï„) remains below its yield stress (Ï„_o) but the fluid exhibits Newtonian behaviour for Ï„ > Ï„_o.

Mathematically, equations 1a and 1b represent this behaviour.

Flow behaviour of several industrially encountered fluids are well represented by the Bingham plastic model (equations 1a and 1b). Liquids like paints, pulp, foodstuffs, liquid chocolate, fresh concrete, water-mud mixtures exhibit viscoplasticity that can be represented by this model.

Only very few studies on hydraulic jump in Bingham plastic fluid have been reported. Ogihara and Miyazawa [2] reported experimental investigations using bentonite mud suspension and Zhou et al. [3] used lubrication theory to analyse jump. Zhou et al. [3] proposed expressions to predict conjugate depths (free surface height just before and after jump), sequent bottom shear stress and critical depth (corresponding to Fr =1). A few studies have analysed the instabilities associated with roll waves for Bingham plastic flow in inclined channels under the action of gravity. In the present study, the shallow water theory, an established simple technique to analyse jump for Newtonian lipids, is adopted to unravel the jump hydrodynamics for thin film Bingham plastic flow in an open rectangular horizontal channel. The analysis predicts steady state free surface profiles and estimates the jump location through mass momentum balance using Rayleigh shock across the jump. The jump strength (ratio of downstream to upstream height) is related to upstream Froude number through the Bélanger equation. Numerical simulations in COMSOL Multiphysics provide additional insight into the jump region, where the theory exhibits a discontinuity.

2. Shallow water analysis

The shallow water analysis is based on the assumption that vertical length scale is much smaller than that of horizontal length scale and pressure is due to hydrostatic head. As a result, horizontal velocity scale outweighs vertical velocity scale. In order to determine the free surface profile, the flow domain is divided into two regions— (i) a sheared region for 0≤y≤h_o where h_o is the liquid height corresponding to τ = τ_o and (ii) a plug region for h_o≤y≤h where, h is the free surface height from the channel floor (Figure 1). In sheared region, the velocity profile of liquid is assumed to be parabolic whereas, the flow velocity in the plug region (u_p) is considered to be uniform vertically but varying along the channel length, i.e., u_p ≠ f(y) but u_p = f(x). The shear stress at the channel floor is maximum and decreases with increase in y till it becomes equal to yield stress at y=h_o (yield surface). Due to different velocity profiles in the sheared and plug regions, momentum balance equation with the assumptions of shallow water theory takes separate forms in these domains. Simplification of these equations result in ordinary differential equations for liquid free surface height with respect to streamwise direction, as represented by equations (2) and (3). Equations (2) and (3) are solved numerically using Runge-Kutta 4^th order method to determine the free surface profile. The jump location and strength are evaluated from free surface profile using Bélanger equation.

3. Numerical Simulation

Numerical simulations are performed with phase-field method in COMSOL Multiphysics 6.0. The finite element method is used by commercial code available with the software. The flow is assumed to occur in an open channel wide enough to neglect effect of side walls, thus rendering flow to be two-dimensional. The two-phase laminar flow model is adopted to account for the air-liquid interactions at the free surface before and after the jump. During numerical simulation with Bingham plastic model, the solution encounters a singularity due to solid type behaviour below yield stress. This is overcome by using Bingham-Papanastasiou regularized model (equations 4a and 4b). The model incorporates additional parameters for a continuous reduction of viscosity exponentially as shown by equations (4a) and (4b) and thus offers a feasible solution from simulation. When , m→∞ the exponential term of equation 4a tends to zero and equations 4a and 4b reduces to Bingham plastic model described by equations 1a and 1b. In the present study, Bingham plastic model is used to derive the analytical solution. To obtain a feasible solution by numerical simulation, the Bingham-Papanastasiou model is employed but with a sufficiently high m (m =1000) which facilitates a fluid flow behaviour similar to that offered by a Bingham plastic fluid.

4. Validation of theoretical analysis and numerical simulation

The free surface profiles obtained from shallow water theory and numerical simulation are presented in Figure 2 for constant fluid physical properties and τ_o . Figures 2(a) –(c) show the profiles at three different liquid inlet velocity, u_inlet= 1, 1.2 and 1.4 m/s respectively. The Figure 2 shows a fair agreement between the free surface profiles predicted from theory and simulations both upstream and downstream of the jump. The mismatches that arise can be attributed to the assumptions of self-similar velocity profiles upstream and downstream of jump and a constant thickness of the plug flow region. In reality, the velocity profile changes considerably due to formation of vortex at jump region resulting in considerable changes in the thickness of plug region especially at jump vicinity.

5. Parametric variations

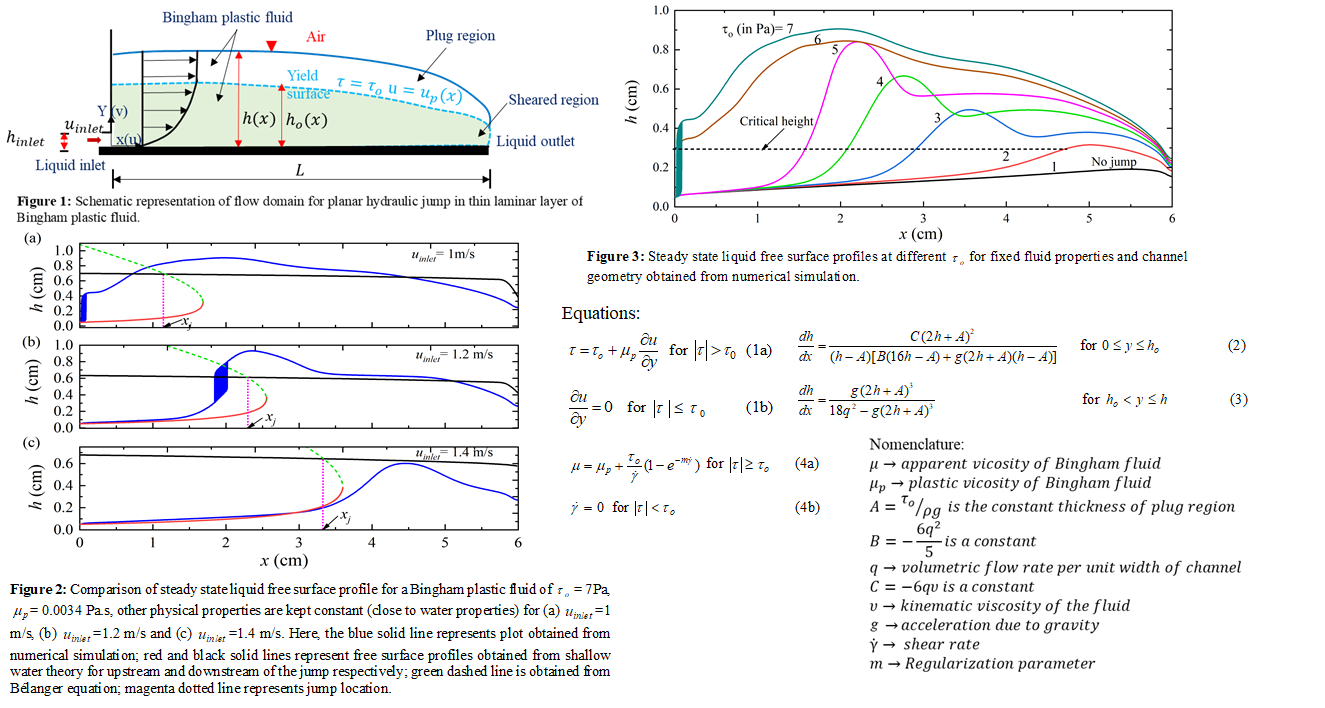
The study presents similar influence of geometric and flow parameters for Bingham plastic and Newtonian liquids. However, the yield stress is noted to have a significant effect on jump formation. The jump location shifts towards the channel inlet and jump strength increases with increase in yield stress (Figure 3) when all other flow parameters and fluid physical properties except Ï„_o are kept constant. Figure 3 also reveals that variation of Ï„_o does not influence the upstream profile while the downstream profile changes considerably.

6. Conclusion

The study provides an in-depth investigation of planar laminar hydraulic jump in Bingham plastic liquids. We propose analytical expressions for the steady state free surface profile, jump location and jump strength for flow in an open rectangular horizontal channel. The shallow water analysis incorporates two different expressions (equations 2 and 3) for the sheared and plug regions to obtain the upstream and downstream profiles. The analytical results are in close agreement with numerical simulation. Numerical simulation gives addition insights of jump where the theory fails to do so. The study reveals the significant influence of yield stress on jump formation.

References

1. Dhar, M., Das, G. & Das, P. K. 2020 Planar hydraulic jumps in thin film flow. of Fluid Mech. 884, 1–26.
2. Zhou, J. G., Shu, J.-J. & Stansby, P.K. 2007 Hydraulic jump analysis for a Bingham fluid. Hydraul. Res. 45, 555–562.
3. Ogihara, Y. & Miyazawa, N. 1994 Hydraulic characteristics of flow over dam and hydraulic jump of Bingham fluid. Jpn. Soc. Civil Eng. 485, 21–26 (in Japanese).