(424b) Growth Modeling for Morphology Predictions of Organic Molecular AB Crystals

Industrial crystals are normally grown at temperatures and supersaturations conducive to layered growth mechanisms such as spiral growth or 2D nucleation. Such regimes ensure gradual addition of growth layers with low impurity uptake. On the atomic scale, growth of crystal layers occurs via attachment of growth units (e.g., monomer, molecules, dimers, etc.) mainly at kink sites. Attachment can also occur on an edge site forming an adatom, or at a step vacancy (pit), thereby forming or destroying kinks, respectively. Gradual incorporation of growth units at various sites along an edge propels it in the normal direction at a rate called the step velocity. Kink density and step velocity are fundamental parameters influencing crystal growth rates. A new theory of crystal growth modeling step velocity and kink density is successfully applied at predicting the morphology of two API crystal systems namely, a doravirine derivative and celecoxib.

For Kossel crystals, step kinetics and the influence of supersaturation and temperature on kink density and step velocity are well-studied. Several nonequilibrium (NEQ) kink density and step velocity models[1–6] have been proposed for the Kossel case. Padwal and Doherty[7] laid out a simplified steady-state framework (SSSF) accounting for only the most-likely surface events in master equation formulation. Note that Kossel models can be applied to centrosymmetric growth units, owing to the presence of an inversion center in these molecules.

For non-Kossel crystals, few equilibrium kink density models [8–10] and nonequilibrium kink density models[11] have been proposed in the literature. Cuppen et al. [8] proposed a step velocity model and derived equilibrium kink density formulations for various edge structures for AB crystals (molecular crystal with two growth units Z = 2, Z^’ = 1,2) based on broken bond energetics for kink formation. Kuvadia and Doherty proposed an equilibrium kink density and derived a net kink rate formulation for step velocity calculation of n noncentrosymmetric growth units. Tilbury et al. [10] proposed a new equilibrium kink density formulation through characterization of surface energy into terrace, edge and kink energies. They also proposed a step velocity expression accounting for the timescales of reorganization and transformation. These equilibrium kink density models are based on Boltzmann formulations, assuming statistical independence of kink sites. However, as noted by Cuppen et al.[8], for non-Kossel steps, kinks are no longer statistically independent and correlated with the neighboring junctions in order to satisfy configurational constraints imposed by the unit cell. Therefore, the existing equilibrium kink density models can only provide an approximation of kink density at equilibrium for non-Kossel crystals owing to the lack of consideration of spatial correlation between kinks. Chernov[12], Zhang and Nancollas[11], Cuppen et al.[8] proposed step velocity models for AB crystal systems, which Cuppen et al.[8] compared with kMC simulations for various step edges and concluded that none of the models provide accurate description for all the different patterns of step edges of an AB crystal. Hence the AB step kinetics cannot be described by a single step velocity expression, because of the differences in the local step structure.

In this work, we extend the SSSF proposed by Padwal and Doherty[7] for nonequilibrium kink density modeling to the case of AB type crystals with two asymmetric growth units in the unit cell. In SSSF, steady-state analysis is conducted for the predominant junctions (e.g., single and double kinks) accounting for only the most-likely events forming, destroying or transforming the junctions. The majority of surface events have low probability and are neglected to maintain simplicity of the framework. The steady-state equations obtained, are thereby solved simultaneously to obtain nonequilibrium kink densities as a function of supersaturation.

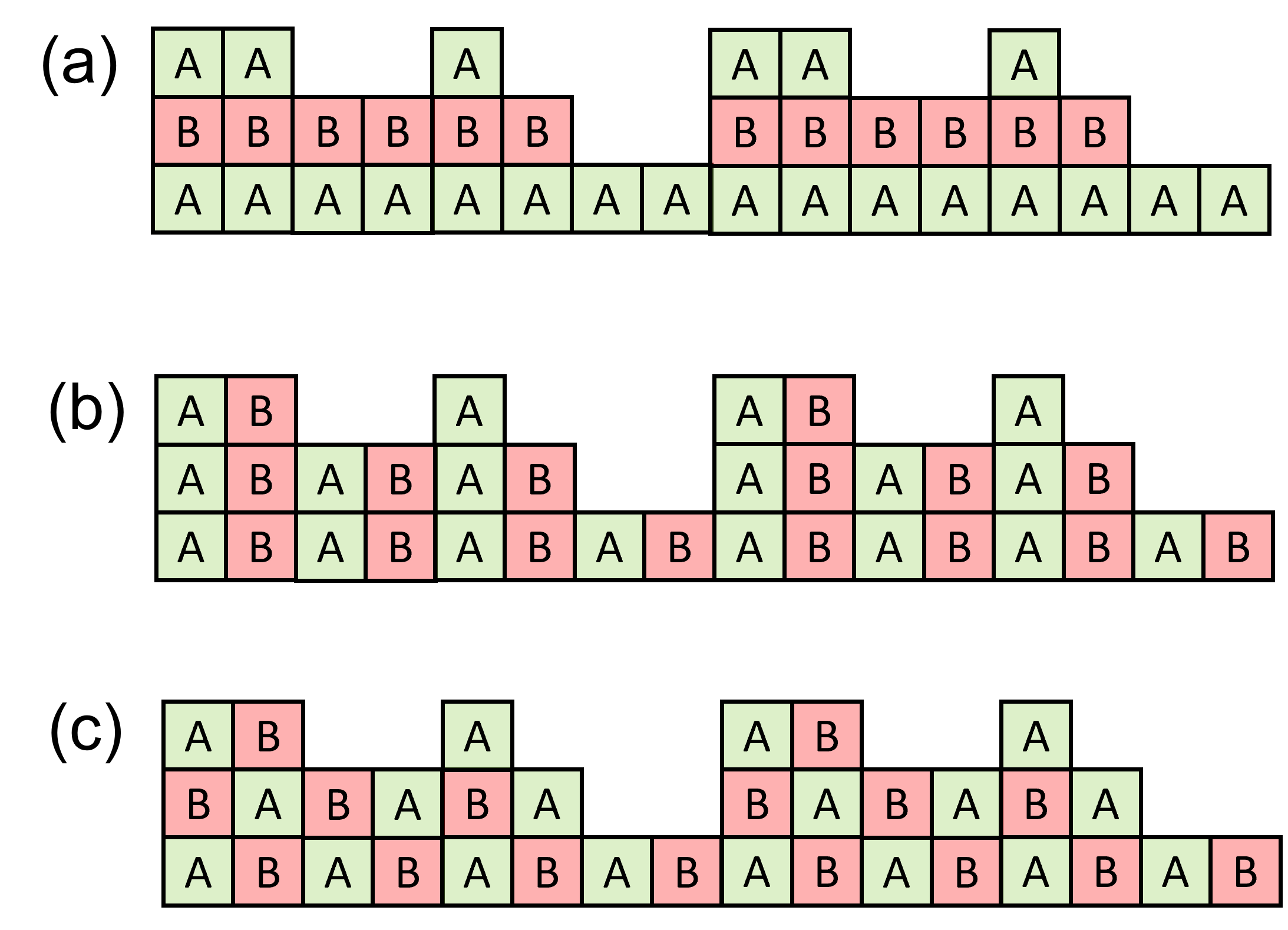
The AB organic crystal has two asymmetric growth units A and B (Z = 2) in the unit cell. The growth units are identical in solution and integrate into the crystal lattice in different orientations. The growth units are asymmetric and do not have an inversion center, resulting in the total number of asymmetric units to be Z'= 1 or Z' = 2. Asymmetry of the growth unit is distinct from asymmetry of the crystalline lattice.[9] Models developed in this work are applicable to asymmetric growth units crystallizing in any space group. For such a crystal system, face configurations with varied patterns of A and B growth units are observed on crystal surfaces. Different face configurations in totality generate three kinds of step edges as demonstrated in Fig. 1. Different steps constitute different kink types and undergo different surface processes resulting in different step kinetics and must be treated individually. Application of SSSF to each of the edge structures yields nonequilibrium kink densities. A generalized step velocity model is proposed for each of the step configurations, utilizing kink densities obtained through SSSF.

Such a step velocity model extends the accuracy and applicability of current mechanic growth models to higher ranges of supersaturation. Hence the model can be applied for crystals grown via antisolvent crystallization at high driving forces. The step velocity model is applied to API systems of a doravirine derivative and celecoxib to generate crystal morphologies and compared with experimental shapes. Rapid morphology predictions using such a step velocity model will enable fast exploration of design space for pharmaceutical APIs towards engineering crystals with desired properties.

References:

1. Frank, F. C. Nucleation-controlled growth on a one-dimensional growth of finite length. Journal of Crystal Growth 1974, 22, 233–236.

2. Voronkov, V. V. Movement of an elementary step by means of formation of one-dimensional nuclei. Soviet Physics Crystallography 1970, 15, 8.

3. Zhang, J.; Nancollas, G. H. Kink densities along a crystal surface step at low temperatures and under nonequilibrium conditions. Journal of crystal growth 1990, 106, 181–190.

4. Cuppen, H. M.; Meekes, H.; van Veenendaal, E.; van Enckevort, W. J. P.; Bennema, P.; Reedijk, M. F.; Arsic, J.; Vlieg, E. Kink density and propagation velocity of the [010] step on the Kossel (100) surface. Surface Science 2002, 506, 183–195.

5. Van der Eerden J. P. In Handbook of Crystal Growth, Vol. 1, J., H. D. T., Ed.; Elsevier: Amsterdam, 1993; Chapter 5, p 307.

6. Joswiak, M. N.; Peters, B.; Doherty, M. F. Nonequilibrium Kink Density from One-Dimensional Nucleation for Step Velocity Predictions. Crystal Growth & Design 2018, 18, 723–727.

7. Padwal, N. A.; Doherty, M. F. Simple Accurate Nonequilibrium Step Velocity Model for Crystal Growth of Symmetric Organic Molecules. Crystal Growth & Design 2022.

8. Cuppen, H. M.; Meekes, H.; van Enckevort, W. J. P.; Vlieg, E. Kink incorporation and step propagation in a non-Kossel model. Surface Science 2004, 571, 41–62.

9. Kuvadia, Z. B.; Doherty, M. F. Spiral Growth Model for Faceted Crystals of Non-Centrosymmetric Organic Molecules Grown from Solution. Crystal Growth & Design 2011, 11, 2780–2802.

10. Tilbury, C. J.; Joswiak, M. N.; Peters, B.; Doherty, M. F. Modeling Step Velocities and Edge Surface Structures during Growth of Non-Centrosymmetric Crystals. Crystal Growth & Design 2017, 17, 2066–2080.

11. Zhang, J.; Nancollas, G. H. Kink density and rate of step movement during growth and dissolution of an AB Crystal in a nonstoichiometric solution. Journal of Colloid and Interface Science 1998, 200, 131–145.

12. Chernov, A. A.; Rashkovich, L. N.; DeYoreo, J. J. In AIP Conference Proceedings, 2007; Vol. 916, pp 34–47.