(535e) Phase Equilibria and Relative Permittivity in Fluid Mixtures with Co-Oriented Fluid Functional Equation for Electrostatic Interactions (COFFEE) and Molecular Simulations

Knowledge of thermophysical properties of fluids and fluid mixtures is essential for the successful design and operation of separation equipment used in chemical engineering applications. It is therefore important to have tools that can predict these properties accurately, especially for mixtures, where experimental data are typically scarce. One essential characteristic of a given fluid mixture is its vapor-liquid equilibrium (VLE), which is of central importance e.g. in distillation processes. For industrial applications, models for the excess Gibbs energy (g^E-models) are typically used to describe and predict phase equilibria. However, g^E-models depend heavily on binary mixture data to achieve accurate correlations of VLE. A more theoretical approach that can be used to even predict phase equilibria is the use of thermodynamic equations of state (EOS). The SAFT family of EOS [e.g. 1-6] approach in particular has been used extensively in both industry and academic research. However, making accurate predictions for mixtures often still proves challenging when the mixtures’ constituents differ substantially in terms of their polarity, i.e. when non-polar components are mixed with strongly polar ones or when associating polar components are mixed with non-associating polar ones. One reason for these challenges is that association and polar interactions are usually considered through separate contributions to the free energy, although these phenomena are intrinsically linked through their (at least partly) electrostatic nature. That is, associating substances always also exhibit polar interactions although any given polar substance may or may not exhibit association [7]. EOS face additional challenges when used to describe electrolyte solutions. To evaluate ionic interactions with Coulomb’s law, the relative permittivity is required. However, the relative permittivity is typically not accessible by EOS and must be calculated with auxiliary models [8].

The Co-Oriented Fluid Functional Equation for Electrostatic interactions (COFFEE) [9] seeks to address both of these challenges [9,10]. To achieve this, the contribution to the free energy due to electrostatic interactions is formulated as a functional of the orientation distribution function (ODF), which offers a statistical description of the intermolecular orientational structure in the fluid. This is a unique feature for an EOS, which links different but related kinds of electrostatic interactions in a natural way. Through the ODF, the relative permittivity including orientation polarization also becomes directly accessible via Kirkwood’s equation. COFFEE has already been used successfully to describe the VLE of simple polar model fluids and simple mixtures, as previously shown at the AIChE annual meeting. It has also been employed to model the VLE of hydrogen chloride, showing improvements over PC-SAFT-like approaches [9]. Furthermore, the relative permittivity of polar model fluids has been predicted successfully and compared to a variety of other methods [8,10,11].

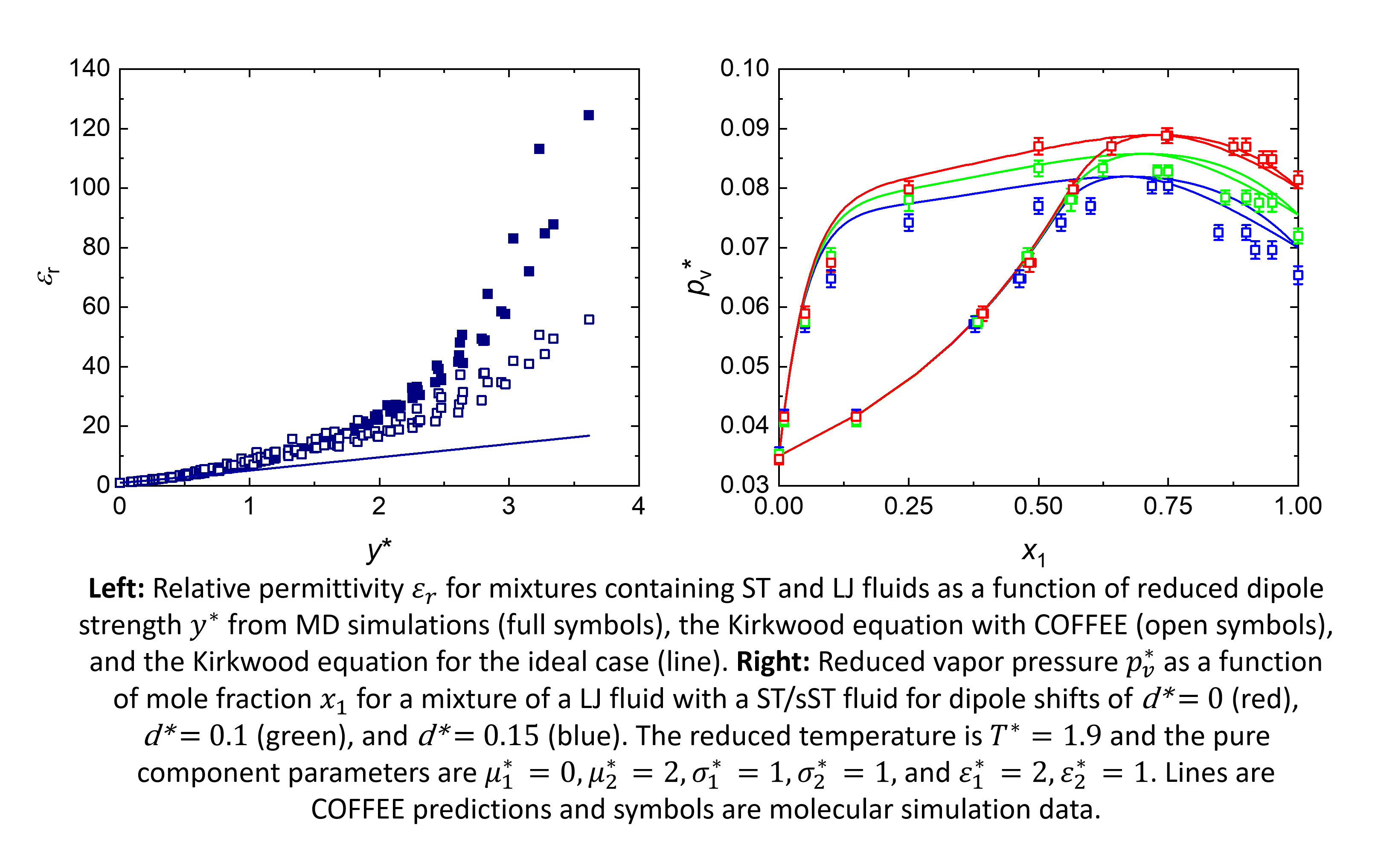
In this contribution, the study of mixtures is further extended. Using COFFEE’s prediction for the ODF in mixtures in combination with Kirkwood’s equation, the relative permittivity is predicted for a wide variety of binary mixtures containing Lennard-Jones (LJ) and Stockmayer (LJ + point dipole, ST) fluids. The results from COFFEE are compared to new results calculated with molecular dynamics (MD) simulations conducted with the molecular simulation software ms2 [12]. Results from COFFEE and MD are in good agreement. Only qualitative agreement is found at high dipole strength, where COFFEE underestimates the relative permittivity quantitatively, but still outperforms Kirkwood’s equation without orientation polarization. An overview of the results is given in the attached figure (left), where the results for the permittivity from COFFEE and MD and the ‘ideal’ case, for which the angular correlation is neglected within Kirkwood’s equation, are plotted as a function of the dipole strength for a variety of mixtures.

Previously presented results for the VLE of mixtures containing LJ and ST fluids are extended to include binary mixtures containing shifted ST fluids (sST), for which the point dipole is shifted away from the LJ center along its axis. COFFEE is uniquely able to make predictions for such fluids with decentral dipoles. These fluids exhibit an orientational structure typical of associating fluids, which is considered via the incorporation of the ODF into the theory. Results from COFFEE compare favorably to results from Monte-Carlo (MC) simulations [13] conducted with ms2 for a large variety of different mixtures. The impact of the dipole shift on saturated densities and vapor pressure is predicted correctly by COFFEE, but somewhat underestimated for the latter, which becomes apparent at high sST contents. Exemplary results for a nonpolar-polar mixture are shown in the attached figure (right).

Finally, predictions for the VLE of binary mixtures of simple real fluids, including associating and non-associating polar fluids, are presented and evaluated. Avenues for the further development of COFFEE and its application to more complex fluids are discussed briefly.

[1] W. G. Chapman, K. E. Gubbins, G. Jackson, M. Radosz, New Reference Equation of State for Associating Liquids, Ind. Eng. Chem. Res. 29 (1990) 1709-1721.

[2] S. P. Tan, H. Adidharma, M. Radosz, Recent Advances and Applications of Statistical Associating Fluid Theory, Ind. Eng. Chem. Res. 47 (2008) 8063-8082.

[3] C. McCabe, A. Galindo, SAFT Associating Fluids and Fluid Mixtures, Royal Society of Chemistry, Cambridge, 2010.

[4] C. O. Emborsky, Z. Feng, K. R. Cox, W. G. Chapman, Recent advances in classical density functional theory for associating and polyatomic molecules, Fluid Phase Equilibr. 306 (2011) 15-30.

[5] J. Gross, G. Sadowski: Perturbed-Chain SAFT: An Equation of State on a Perturbation Theory for Chain Molecules, Ind. Eng. Chem. Res. 40 (2001) 1244-1260.

[6] J. Gross, G. Sadowski, Application of the Perturbed-Chain SAFT Equation of State to Associating Systems, Ind. Eng. Chem. Res. 41 (2002) 5510-5515.

[7] E. Arunan, G. R. Desiraji, R. A. Klein, J. Sadlej, S. Scheiner, I. Alkorta, D. C. Clary, R. H. Crabtree, J. J. Dannenberg, P. Hobza, H. G. Kjaergaard, A. C. Legon, B. Mennucci, D. J. Nesbitt: Defining the hydrogen bond: An account (IUPAC Technical Report), Pure Appl. Chem. 83 (2011) 1619-1636.

[8] M. Kohns, J. Marx, K. Langenbach: Relative Permittivity of Stockmayer-Type Model Fluids from MD Simulations and COFFEE, J. Chem. Eng. Data 65 (2020) 5891-5896.

[9] K. Langenbach: Co-Oriented Fluid Functional Equation for Electrostatic interactions (COFFEE), Chem. Eng. Sci. 174 (2017) 40-55.

[10] K. Langenbach, M. Kohns: Relative Permittivity of Dipolar Model Fluids from Molecular Simulation and from the Co-Oriented Fluid Functional Equation for Electrostatic Interactions, J. Chem. Eng. Data 65 (2020) 980-986.

[11] M. Kohns, J. Marx, K. Langenbach: Critical assessment of perturbation theories for the relative permittivity of dipolar model fluids, Chem. Eng. Sci. 245 (2021) 116875.

[12] R. Fingerhut, G. Guevara-Carrion, I. Nitzke, D. Saric, J. Marx, K. Langenbach, S. Prokopev, D. Celný, M. Bernreuther, S. Stephan, M. Kohns, H. Hasse, J. Vrabec: ms2: A molecular simulation tool for thermodynamic properties, release 4.0, Comput. Phys. Commun. 262 (2021) 107860.

[13] J. Marx, M. Kohns, K. Langenbach: Vapor-liquid equilibria of binary mixtures containing Stockmayer-type model fluids from Monte-Carlo simulations, Fluid Phase Equilibr. 568 (2023) 113742.